Proof of Central Limit Error Scaling for ESPRIT Algorithm

Ties

Summary and 1 Introduction

1.1 Esprit algorithm and Central Limit Error scale

1.2 Contribution

1.3 Related work

1.4 Technical overview and 1.5 Organization

2 Proof of the central limit error scale

3 Proof of optimal error scaling

4 Theory of the disturbance of second -order clean vectors

5 Comparison of strong clean vectors

5.1 Construction of “good” p

5.2 Taylor extension in relation to the terms of error

5.3 Error cancellation in Taylor's expansion

5.4 Proof of theorem 5.1

A preliminary

B Vandermonde matrix

C Delayed proof for section 2

D deferred proof for section 4

E deferred evidence for section 5

F Lower Bound for the spectral estimate

References

2 Proof of the central limit error scale

The main roadmap is the same as previous work [LLF20] With small modifications. All technical evidence and calculations are postponed to Annex C

This result is similar to existing results such as [Moi15, Lem. 2.7] And [LLF20, Lems. 2, 5, & 6] And our proof (in appendix C.2) is based on similar ideas. The main ingredients of our proof of this result are the limits of moitra on the singular values ​​of the Vanderonde matrices (Annex B.1), the standard results in the theorem of the matrix disturbance (Annex A.5) and a lemma of comparison between the Vanderonde and the clean bases (Lemma 1.7)

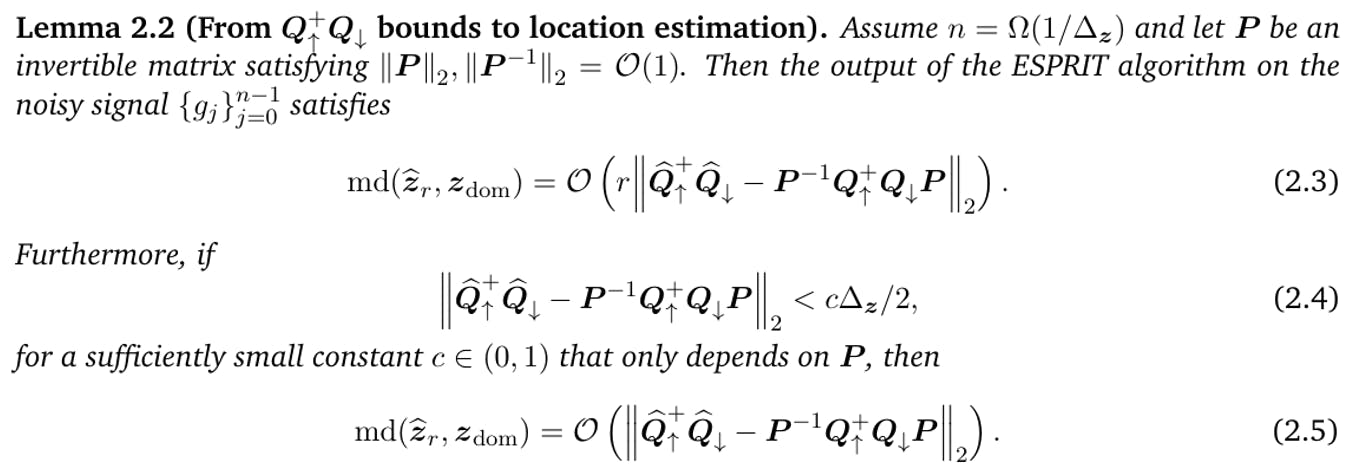
To convert the linked equation. (2.2) in usable information for the spirit algorithm, we use the following result:

This result slightly improves lemma 2 in [LLF20] And is proven by the combination of the Bauer-Fike theorem (theorem A.11) and the resolving approach for the disturbance of the own values ​​(Lemma A.13). The proof is postponed to Annex C.3.